A few words about math

One more unhinged rant before I turn the blog over to the Not Quite Noahpinion super-team.

When I was 15, I had an epiphany that changed my life. I had always been pretty good at math, but I found it boring. Who cared about all these abstract numbers? There was no pleasure in it for me. Even when you calculated the area of a back yard that could be enclosed by a certain length of fence, or whatever, it just seemed like an analogy, designed to make a boring pointless subject more interesting by relating it to something "real". Still boring. Despite teachers' entreaties, I never joined the math team in high school.

Then I took my first physics class. Flipping through the book, I groaned. There was so much boring math! But when I sighed and finally sat down to read the first chapter, the author told me an amazing thing. Math, he wrote, was the "language of nature". It could be used to represent reality. Those abstract numbers on the page weren't just something we made up; they were real things.

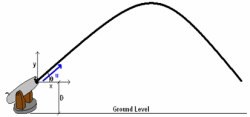
At first I didn't even quite get what he was saying. Then I worked an example, using projectile motion to calculate the range of a cannonball. I suddenly imagined that I was a Turkish gunner, lobbing iron balls at the walls of Constantinople (yes, even then I was a history geek). Not realizing that gunners actually found their range by trial and error until the 19th century, or that the conquest of Constantinople came well before Galileo and Newton, I suddenly thought: "If I could do this simple math, I could hit the wall really accurately! But if I did the math wrong, I'd miss!" All at once, it hit me: Math could predict the future. Math conveyed power.

Math was real.

(Of course, later we confirmed the model's usefulness by predicting - very accurately! - a real metal ball in a real lab. But I was already sold on the concept, because I trusted that the real-world effectiveness of the math had been verified by people in the gunnery business.)

From then on I steadily began to enjoy math more. Eventually I discovered the abstract "beauty" that mathematicians talk about, especially when it came to proofs. But when I did physics math, there was always a special thrill that other math never held for me. It was the idea that I was mastering the real Universe with my abstract mind.

Fast-forward a few years later, and I had left physics behind (despite enjoying it and being good at it). When I entered econ grad school, I expected - naively, it turned out - that the math that people did would be like the math I had done in physics. I expected that economists' models would largely be reliable, well-tested tools for predicting the future, just like I had predicted the cannonball with high school algebra.

And actually, some of the econ math seemed to qualify. Game theory only annoyed me slightly. Though its assumptions weren't often satisfied in the real world, seemed like it would work if we could get the incentives right (and in fact, it very often does, in experiments). Consumer theory was a little more dubious - how could you measure a demand curve in practice? - but choice theory seemed like something that would work if people had stable preferences and you could nail them down empirically. I was a little disturbed by the misuse of the word "axiom" to refer to things that were actually testable (like revealed preference), but I let that one slide.

But macro was a different story.

In macro, most of the equations that went into the model seemed to just be assumed. In physics, each equation could be - and presumably had been - tested and verified as holding more-or-less true in the real world. In macro, no one knew if real-world budget constraints really were the things we wrote down. Or the production function. No one knew if this "utility" we assumed people maximized corresponded to what people really maximize in real life. We just assumed a bunch of equations and wrote them down. Then we threw them all together, got some kind of answer or result, and compared the result to some subset of real-world stuff that we had decided we were going to "explain". Often, that comparison was desultory or token, as in the case of "moment matching".

In other words, the math was no longer real. It was all made up. You could no longer trust the textbook. When the textbook told you that "Households maximize the expected value of their discounted lifetime utility of consumption", that was not a Newton's Law that had been proven approximately true with centuries of physics experiments. It was not even a game theory solution concept that had been proven approximately sometimes true with decades of economics experiments. Instead, it was just some random thing that someone made up and wrote down because A) it was tractable to work with, and B) it sounded plausible enough so that most other economists who looked at it tended not to make too much of a fuss.

We were told not to worry about this. We were told that although macro needed microfoundations - absolutely required them - it was not necessary for the reality of any of these microfoundations to be independently confirmed by evidence. All that was necessary is that the model "worked" after all the microfoundations were thrown together. We were told this not because of any individual failing on the part of any of our teachers, but because this belief is part of the dominant scientific culture of the macro field. It's the paradigm.

Anyway, that was the beginning of my exposure to macro, but not by any means the end. The math got a lot hairier and more kludgey, though not more beautiful. Only occasionally - in a special class taught by Miles Kimball - was there the kind of elegance or deep conceptual math that I had enjoyed in college math classes. Only occasionally - as in the "matching function" of a Diamond-Mortensen-Pissarides labor search model - was there a microfoundation that people actually bothered to check rigorously against reality. Usually, the math was just a whole lot of algebra (yawn) with more made-up stuff. Kreps-Porteus preferences. Heterogeneous agent models. Investment adjustment costs. You would very formally define an "equilibrium" in terms of some functional equations, and you'd stick the system in a computer to solve for you, tossing in parameters from wherever you could grab them ("calibration"). 

So the math in most of the macro papers I read was easy math, implemented in a boring, kludgey, tedious way. That would have been OK if I could have convinced myself that the math represented real stuff like in physics. But mostly, it seemed not to.

It occurred to me then that there were more uses of math than the ones I had been taught about in high school. In addition to being beautiful and representing reality, math can be used to signal intelligence. Economists hold forth on a lot of stuff, and we often tend to listen to the ones we think are smartest. If I can do some tricks that the next guy can't, that can make me seem more like a sage. "First prove you're smart by doing some hard math thing," an economics prof once told me with a grin, "and then you can write about whatever you want." I doubt most profs are so cynical, but the incentive system is certainly there. 

Math can also be used as obscurantism; if every paper in a field starts with a dense thicket of formal statements and functional equations, it will be difficult for even very smart outsiders to come in and evaluate what the people in a field are doing with their time. Again, I doubt all but the most cynical macroeconomists would be intentionally obscurantist; they would just be subtly rewarded for doing things that ended up having an obscurantist result.

Anyway, the thick, sludgy swamp of math-without-beauty-or-truth ended up discouraging me from doing math at all. My dissertation didn't really use anything beyond high-school algebra, and was all about experiments and empirics instead of theory. But I miss math. I miss doing cool, deep, beautiful math for its own sake. But much more than that, I miss doing math that felt like it represented something real.

Luckily, Stony Brook is very strong in quantitative finance, so I'm getting to explore that field more. Despite the, um, well-known dangers of putting quant finance into practice, the math - and its relation to reality - is considerably more to my liking than the stuff I did in grad school.

(Note: The purpose of this post is not to say that economists shouldn't use math, or should use less. Check out this older post to see what I think about that broader question. Here I'm just saying that the math of macroeconomics is unappealing to me personally.)


Update: Paul Krugman points out that macro math is also useful for clarifying your thinking, checking your self-consistency, and exploring the implications of various sets of assumptions. That's all true, of course, and in fact I wrote much the same thing in this post last year. But those things just don't give me the same rush of intellectual excitement that the reality of physics math (or the beauty of pure math) always gave me. Others' mileage may vary.

Also, Paul suggests that my macro teachers might have really believed that their models were The Truth. Although some might have, I think most (I had 8 in all!) simply wanted to give students the technical tools that they thought we needed in order to succeed and publish papers in the macro world. In general I think they did a very good job in that regard.


Update 2: Bryan Caplan thinks that there's too much math in econ, period (and not just macro). He says that "economath" is often just a tedious and unnecessary translation of economic intuition into math-language. Interesting, and worth a read. But again, this is getting a bit far from my point in this post, which is about how fun economath is(n't) for me, not how useful it is for the world.