
Consider Proposition H: "God is watching out for me, and has a special purpose for me and me alone. Therefore, God will not let me die. No matter how dangerous a threat seems, it cannot possibly kill me, because God is looking out for me - and only me - at all times."
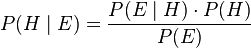
Suppose that you believe that there is a nonzero probability that H is true. And suppose you are a Bayesian - you update your beliefs according to Bayes' Rule. As you survive longer and longer - as more and more threats fail to kill you - your belief about the probability that H is true must increase and increase. It's just mechanical application of Bayes' Rule:
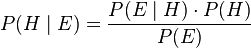
Here, E is "not being killed," P(E|H)=1, and P(H) is assumed not to be zero. P(E) is less than 1, since under a number of alternative hypotheses you might get killed (if you have a philosophical problem with this due to the fact that anyone who observes any evidence must not be dead, just slightly tweak H so that it's possible to receive a "mortal wound").
So P(H|E) is greater than P(H) - every moment that you fail to die increases your subjective probability that you are an invincible superman, the chosen of God. This is totally and completely rational, at least by the Bayesian definition of rationality.
So P(H|E) is greater than P(H) - every moment that you fail to die increases your subjective probability that you are an invincible superman, the chosen of God. This is totally and completely rational, at least by the Bayesian definition of rationality.
If you think this is weird, consider a closely related question: Suppose H was true, and you were an invincible God-protected superman. How would you know? Simple: you'd go get in a bunch of dangerous situations, and when you failed to die, you'd gradually realize the truth. The more dangerous the situations you chose, the faster the truth would be revealed - if you jumped in front of a train and failed to die, that would be stronger evidence than if you just drove to the store without a seat belt and made it back safely.
But nevertheless, every moment contains some probability of death for a non-superman. So every moment that passes, evidence piles up in support of the proposition that you are a Bayesian superman. The pile will probably never amount to very much, but it will always grow, until you die.
So does this mean that any true Bayesian will eventually accept invincibility as his working hypothesis? No. There are other hypotheses that explain your failure to die just as well as H does, and which are mutually exclusive with H. The ratio of the likelihood of H to the likelihood of these alternatives will not change as you continue to live longer and longer. But this gets into a philosophical thing that I've never quite understood about statistical inference, Bayesian or otherwise, which is the question of how to choose the set of hypotheses, when the set of possible hypotheses seems infinite.
Anyway, it seems to me that "Bayesian superman" is not just a prescriptive definition of how a rational person reacts to his own failure to die, but also a description of the observed behavior of teenagers. Teenagers - especially boys - are commonly described as "thinking they're invincible." Often this belief noticeably decreases when a young man sustains a major injury, witnesses a major injury or death, or has a close call with death. This, I suspect, is because most people's belief in their own invincibility is a little more complex than the "Proposition H" I've written above - it includes things like "God is looking out for my friends," and "God will stop me from getting the s*** kicked out of me," etc. A lot of people seem to start life with a non-negligible belief that this kind of special protection is watching over them. Sadly, they all eventually learn otherwise.
God is watching out for me, and has a special purpose for me and me alone. Therefore, God will not let me die.
ReplyDeleteMay be the special purpose is accomplished through the death - while He is watching!
OK, at the risk of appearing a new-age whack-a-doodle, there may be a way out of this. It has been said that one of the goals of Buddhism is, "the destruction of all speculative views." So, the "self" is a view, an abstract idea. Is it a reality? If it isn't real then there is nothing besides the body which dies. The body is merely another ephemeral phenomena in a world of ephemeral phenomena.
ReplyDeleteConversely, and this is also an idea and as such to be taken metaphorically rather than literally, it has been said by some contemporary mystics that the "true" (and Universal) self is Awareness, which is beyond form and therefore beyond birth and death. So there appears to be the possibility that we are both mortal and immortal, both ephemeral and beyond space & time.
Having said that, I need to grab a turkey sandwich.
Noah,
ReplyDeleteI hope I'm not being a dummy here, as the people who would tell me so can be so cruel, but I think you may have gone wrong in the second to last paragraph, and actually we should become more confident of our invincibility as we get older.
Think of the following pairwise incompatible hypotheses: "I will never die", and "I will die in year Y" for each Y>2013. These are all mutually incompatible, but at the end of 2014 my credence in "I will die in year 2014" should decrease to 0, and my credence in all the others should increase at its expense. I guess the ratios between your credences in the remaining hypotheses will remain the same, other things being equal, but they'll all increase at the expense of the hypotheses getting ruled out. This includes the first hypothesis, that you're invincible. So a Bayesian should get more and more confident in their own invincibility, other things being equal.
Increased confidence can still be very small. If you assign a very small prior probability to H, like 1e-10, posterior probability will remain negligible under any conceivable scenario. Even if something like https://www.youtube.com/watch?v=fHG4fPkGpDM happens
DeleteI am a critic of the naïve use of Bayes' rule, but here I think I could defend Bayes.
DeleteMathematically, there seems to be a technical issue with the set of hypotheses. It is well known that the choice of coordinate system for a physical space can affect Bayesian inference. In Noah's example if one takes two hypotheses 'I will never die' and 'I will die some time' then the second hypothesis is compound so using the generalized likelihood (as per Good) one finds that the posterior probability of never dying does not increase the longer you survive.
The problem with considering hypotheses such as 'I will die at age 45' using Bayes' rule naively is that one has to use some probability distribution for the time of death, so one is not comparing 'I will never die' with 'I will die sometime', but 'I will never die' with 'I have a typical life expectancy'. These two do not fill the space, hence Noah's odd result.
In my case, just when I started thinking about this kind of stuff, I got sent off to school where I was a little kid mixed in with older, bigger kids which kept me from thinking that I was invincible. Gradually I became one of the older, bigger kids. At that point, thoughts of invincibility began to arise. Each year they did increase as predicted by Dr. Smith's hypothese till I reached the zenith of teenaged invulnerability. And then I got taken down a notch.
ReplyDeleteNow, in my pre-dotage, each day brings another ache or pain. Mortality seems more certain each day.
Bayesian analysis works pretty good if you choose realistic priors.
I know that. I read 'believe more and more in his own invincibility' as 'have a constantly increasing degree of belief in his own invincibility'. But I might have been wrong to read it that way; I agree that a Bayesian could (even other things being equal) remain very skeptical about their own invincibility. "The pile will probably never amount to very much, but it will always grow, until you die," as Noah says in the post, immediately before the bit I complained about.
ReplyDeleteIs that Bob Murphy in the photo?
ReplyDeletehehehe
DeleteYeah you witness other kids with beliefs of invincibility turn out to be not-so-invincible and so adjust your belief accordingly.
ReplyDeleteI really am confused by your point here. Further, Bayesian analysis is just a framework, lets not forget. The benefit comes from a willingness to trade some (i.e. a small amount) of bias for efficiency. In the end though, crappy evidence or a crappy prior produces a crappy result (crap in crap out). You could even use non informative priors (i.e. uniform) and place all of the weight on the evidence. Or you can place all of the weight on the prior and little on the evidence.
ReplyDeleteIf you have a strong prior that your superman, your an idiot. Further, if your evidence is not wearing a seatbelt and not dieing, your and idiot, Bayesian logic or otherwise. Bayesian analysis doesnt free you from needing to collect good evidence or form well structured priors. What are you trying to say here?
This comment has been removed by the author.
DeleteThis sounds like Hempel's paradox: the hypothesis "all ravens are black" is apparently supported by a white telephone, since a white telephone is not black, and conveniently not a raven either.
ReplyDeleteThis isn't as paradoxical as it sounds, I think.
ReplyDeleteEven generalising H enormously to "I am immortal", most people never see very much evidence in favour of H. For example, a US male has perhaps a 15% chance of survival to age 90, so by age 90 would have accumulated only about 7:1 evidence in favour of the hypothesis, and the posterior odds would be only about 7 times the prior odds. Once you get to 130 or so, the likelihood ratio starts getting much more impressive, but so it should.
Secondly, someone who believed precisely H would see weak evidence in favour of it over a typical lifetime, but you'd expect a rational person who believed H to also believe a bunch of other weird things, and see strong evidence against some of these things, and so the updating process would be rather more complicated, depending on how the beliefs were related. A real person who believed H probably wouldn't be convinced by evidence on other related topics, but we're assuming a rational Bayesian reasoner -- it wouldn't be paradoxical if an irrational person ended up with strange beliefs.
You could invoke Hume and argue that there is no evidence for H vs "I am immortal precisely up to today", or invoke Hempel's paradox, but I don't think it's necessary to go that far in this case. It is in philosophy of science, but not here.
Note that on the Bayesian's view, the Hempel paradox isn't a paradox. A white telephone really does move the posterior mass towards "all ravens are black". Similarly, your response to this problem is that it isn't really a problem. The posterior mass moves, but not enough to convince any reasonably minded person. The question is whether it should in principle move the posterior mass at all.
DeleteMy understanding of the psychological literature is that the young and old have the same assessments of risk. Where they differ is that the young are much more attuned to the rewards of risky behavior.
ReplyDeleteIf you see someone playing it safe, it's probably because they are on path to "winning" the game - whatever their personal win condition is. If someone's taking big risks, is probably because they're on a path to lose, and need lucky rolls to win anyway.
DeleteThis is a really simple observation that is all to absent in discussions about why the young are as they are, or why men are as they are.
Proposition H also cures hemorrhoids.
ReplyDeleteBest comment.
DeleteMinor correction -- it cures homorrhoids. Not quite sure what they are, and I don't don't want to speculate much (human sterrhoids?), but if we map "e" --> "o" in one word, consistency or accuracy requires the same in the other word, eh?
DeleteNo:
Deletehttp://www.mayoclinic.org/diseases-conditions/hemorrhoids/basics/definition/con-20029852
Only an economist could love this example.
ReplyDelete"How do you know that you are immortal?"
"I have not died because God hasn't provided my purpose yet."
"I'm sorry to be rude because this is a fatal injury, but doesn't it disprove your belief?"
"Not at all. I have fulfilled God's mission."
"What the @#$#$? Why would you say that?"
"Because I'm dying, huhuhu."
The weird thing is that all people actually may be "immortal" - think quantum immortality. One just has to realize that there may be "worse" things than death, so in the end you still have strong incentives not to expose yourself to a risk of injury.
ReplyDeleteLet us not forget that the Bayesian would be vindicated the moment the subject dies...
ReplyDeleteThe problem here is that the true Bayesian would understand that he himself is not special, and so therefore his prior must be based not on solely his own experiences, but on the likelihood of death based on past experiences of everyone. So the Bayesian would look at statistics of wearing a seatbelt and say "I better wear my seatbelt".... they wouldn't simply say "I've never worn my seatbelt and I'm still alive, therefore I don't need to wear my seatbelt."
ReplyDeleteAnother consequence of recognizing that a Bayesian would never use solely their own experience to form their prior is that every bayesian is forced to accept that the probability of eventual death is 1.
Any Bayesian who would ignore all other experiences except their own is no real bayesian at all.
Teenagers don't imagine god shielding them from risks. They simply underestimate risks.
ReplyDeleteUnfortunately, your logic is flawed. First of all, we are dealing here with subjective probabilities. In reality, P(H) =0, so the analysis fails. But even if we accept the use of subjective probabilities, all you have shown is that the subjective probability P(E|H) > P(H). You have not shown that P(E|H) increases with each successive non-death. In fact, you make the classic "gambler's fallacy" mistake. You assume that past events (i.e. not dying in a number of dangerous occasions) affect the probability of future events. This is like saying that because a fair coin toss came up as five heads in the past, the probability of heads is higher on the subsequent toss.
ReplyDeleteWell, clearly "I have lived so far, therefore I will live forever" has a logic flaw but I think you are incorrect to attribute it to the gamblers fallacy. Essentially, the purported Bayesian superman is using his experience to try to determine whether the coin really is fair or if it is weighted in his favor. You're correct that a coin toss leading to five heads in a row shouldn't lead one to bet the farm on the sixth coming up heads, but how about after 100 heads in a row? If the proposition we are testing is "This coin is rigged to strongly favor heads", then our confidence in the assertion should indeed increase as the number of heads in a row increases. Is that really so different from the proposition that "my life is rigged to strongly favor my survival.? Granted, you'd want to give it a lot more then 100 tests before jumping off a bridge ...
DeleteConfidence is a parameter in the equation that determines the number of heads in a raw needed to declare your coin biased.
DeleteEven worse, like many Baysian economists, he confused events with their probabilities.
DeleteAmplifying another comment, epistemologically the Bayesian Statistical Method is only a process for quantifying one's ignorance about a specific process.
ReplyDeleteThe author states: "But this gets into a philosophical thing that I've never quite understood about statistical inference, Bayesian or otherwise, which is the question of how to choose the set of hypotheses, when the set of possible hypotheses seems infinite."
The set of possible hypotheses in ANY system of inference (whether it be classical statistics, the reading of tea leaves, metaphysical dogmatic systems (such as formal religions), measurement based dogmatic belief systems (such as science), consulting of oracles or bayesian statistics) is infinite because the set of possible outcomes in the world is infinite.
So in the realm of statistics: classical method involves performing an experiment, collecting data at the end, performing tests of which 99% of practitioners haven't a clue what the underlying assumptions are, publishing standardized results (but only if they support your claim) which everyone understands but epistemologically shed little light on the topic researched. Classical conveniently ignores outliers to make it easier on the researcher. The trap here is that if your t-test is high the researcher then makes the epistemological leap that they've learned something new because they rejected the null hypothesis.
With Bayesian Statistics the researcher must have a model and then with some rigor state their underlying assumptions before starting to collect data. As the data comes in outliers will be the most significantly weighted and the model will need constant updating. At the end of the experiment the researcher will have a better idea of their uncertainty around a certain process - but they will ALWAYS have uncertainty (just like humans do about anything in reality).
Classical stats are perfect for writing scholarly papers. Bayesian stats are good for taking risk.
p.s. The superman model in the article is simply a bad prior based upon a fallacy and ignoring a lot of relevant information.
Ah ha. My prior, that a windbag would hold forth in these comments, is justified.
ReplyDeleteI don't see anything wrong with the reasoning described in this post.
ReplyDeleteThere is a mistake in defining E to simply be "not being killed" as time passes. This is true for every normal creature. To be a superman the evidence would need to be more like "not being killed in circumstances when ordinary beings would have been killed."
ReplyDeleteVery unlikely you would observe evidence of that over time. You built in the result you wanted by your choice of E.
Noah,
ReplyDeleteTesting H requires a tug of war between confirmative evidence, such as "I am not dead so far" and disconfirmative evidence, such as "all living things die". In this case the latter evidence is conclusive, thus proving H false. Just looking at confirmative evidence to test a hypothesis is an instance of the Confirmation Bias.
What is your conclusive evidence for 'all living things die'?
Deleteuh?
DeleteI think this is very similar to Illusion of Control Bias, and that happens to financial market people all the time. I made money on a trade => I’m a trading genius. That is why so many people quit their jobs to become day traders during the dot com bubble. Everybody looks like a genius when the market is rising.
ReplyDelete"Consider Proposition H: "God is watching out for me, and has a special purpose for me and me alone. Therefore, God will not let me die."
ReplyDeleteI just talked to him and he denied that.
Econometrix: excellent point
ReplyDeleteMassimo uncovered the strawman
ReplyDeleteI would be much more inclined to accept Bayseian Rationality if I wasn't an Orthodox Solipsist.
ReplyDeletefrom the perspective of the superman: how is this problem different from the black swan?
ReplyDeleteIf you perform the same analysis, but with H representing the proposition "I will live to 100", then the same conclusion is reached (every bit of evidence I see increases the belief that I will live to 100), but in that case it seems entirely reasonable.
ReplyDeleteThe difference between the two hypotheses is that our prior belief that we will live to 100 is probably going to be much higher than our prior belief that we are invincible supermen. This means that out posterior belief that we are invincible supermen will only increase very slowly as we fail to die, but our posterior belief that we will live to 100 will rise rather faster.
It seems to me that the Baysian approach here is doing the right thing, *provided* our prior beliefs are reasonable. Am I missing something?